This item is taken from IGCSE Mathematics (0580) Paper 41 of October/November 2012.
To deal with this item, let us summarize the details of the problem:
(a) Syllabus area: Trigonometry
(b) Specific topic: Problems Involving Three Dimensional Figures
(c) Formula Needed:
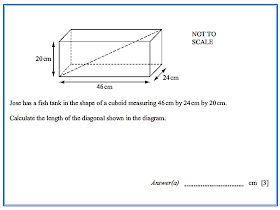
To start with, let us draw a diagonal of the base of the cuboid.
Take note that using the diagonal, we have formed two congruent right triangles at the base, where the shortest side measure 24 cm and the longer side measures 46 cm.
To find the length of the diagonal (hypotenuse), represented by c, let us use the PYTHAGORAS THEOREM.
The diagonal that we have drawn is also a side of another triangle. The longest side (hypotenuse) of this triangle is the diagonal of the cuboid. The shortest side is equal to the height, which is 20 cm, of the cuboid.
Using Pythagoras theorem, the length of the diagonal of the cuboid can be calculated.
Therefore, the length of the diagonal of the cuboid is 55.6 cm.
---------------------------------------------------------------------------------------------------------------
Your comments and suggestions are welcome here. You may write them in the comment box below.
Thank you and God bless!
To deal with this item, let us summarize the details of the problem:
(a) Syllabus area: Trigonometry
(b) Specific topic: Problems Involving Three Dimensional Figures
(c) Formula Needed:
To start with, let us draw a diagonal of the base of the cuboid.
Take note that using the diagonal, we have formed two congruent right triangles at the base, where the shortest side measure 24 cm and the longer side measures 46 cm.
To find the length of the diagonal (hypotenuse), represented by c, let us use the PYTHAGORAS THEOREM.
The diagonal that we have drawn is also a side of another triangle. The longest side (hypotenuse) of this triangle is the diagonal of the cuboid. The shortest side is equal to the height, which is 20 cm, of the cuboid.
Using Pythagoras theorem, the length of the diagonal of the cuboid can be calculated.
Therefore, the length of the diagonal of the cuboid is 55.6 cm.
---------------------------------------------------------------------------------------------------------------
Your comments and suggestions are welcome here. You may write them in the comment box below.
Thank you and God bless!







No comments:
Post a Comment